The next prime number
THE PRIME NUMBER SERIES 23
The next prime number
Let U be an odd number. The next prime number is sought.
This can be done by first determining the number of prime numbers up to U and then the first prime number following U. I split the procedure into two steps because one step would put too much strain on computers.
![]() is the number of prime numbers up to and including the odd number U. Then the following applies:
is the number of prime numbers up to and including the odd number U. Then the following applies:
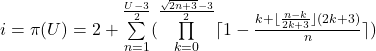
and
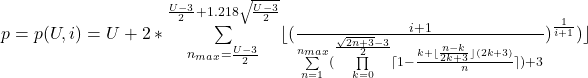
valid for U ≥ 5 ∧ U ![]()
Examples:
Let U=5. Subinserted into the formula for ![]() results in i=3. I.e. up to and including the number 5 there are three prime numbers. And indeed: they are the prime numbers 2, 3 and 5. Thus, the next following prime number p can be calculated with p(5,3)=7. And 7 is actually the first larger prime number after 5.
results in i=3. I.e. up to and including the number 5 there are three prime numbers. And indeed: they are the prime numbers 2, 3 and 5. Thus, the next following prime number p can be calculated with p(5,3)=7. And 7 is actually the first larger prime number after 5.
Let U=713. Inserted into the formula for ![]() , i=127. I.e. up to and including the number 713 there are 127 prime numbers. Thus, p can be calculated as p(713,127)=719.
, i=127. I.e. up to and including the number 713 there are 127 prime numbers. Thus, p can be calculated as p(713,127)=719.
Tested with my prime number test
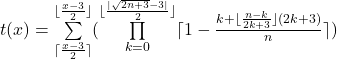
valid for ![]() ≥ 4 ∧
≥ 4 ∧ ![]()
![]()
results in the following values:
t(713)=0 d.h. 713 is not prime
t(715)=0 specifically 715 is not prime
t(717)=0 specifically 717 is not prime
t(719)=1 specifically 719 is prime
This proves that 719 is the first prime number that follows the odd number 713.
Munich, 21 April 2024
Gottfried Färberböck


